
This gives us our simplified formula as A = bh + 3bL. 2.) We can use this to replace (s1 + s2 + s3) in the formula with 3b. Solution: 1.) Since an equilateral triangle is made of three equivalent side lengths, we know that our s1 = s2 = s3. Problem 2: If we are given a triangular prism that has a base formed by an equilateral triangle, how can we simplify the surface area formula before solving it? Equivalently, it is a polyhedron of which two faces are parallel, while the surface normals of the other three are in the same plane (which is not necessarily. A = 123.31 4.) The surface area of the right-angled triangular prism is 123.31. The formula to find the volume of a triangular prism is, Volume base area × length of the prism, which shows the relationship between the area of a triangle. Using the volume of the triangular prism formula, The volume of the given triangular prism base area × length of the prism 56 × 10 560 ft 3. 3.) Now let’s plug our known values into the surface area formula. The base of the triangle (b) 14 ft, and its height (h) 8 ft. Lateral Area 30 cm2 volume36 unit3 Find the surface area and volume of the.
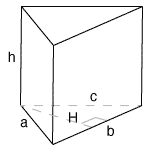
This resource is part of the Math at the. Question: Find the lateral area and volume of the right triangular prism. Using the Pythagorean theorem, we get: (s3) 2 = 4 2 + 7 2 s3 = 8.062. Prior exposure to triangular prisms and to the notion of surface area is helpful. In a right triangular prism, there are 3. 2.) We are still missing s3, which is the hypotenuse of the right triangle. Before you find the surface area of a right triangular prism, you have to know what kind of shape you are dealing with. Step 3: So, the volume of triangular prism is calculated as, V 100 × 3 300 cu. Substitute the given value of base area and length in the formula. Step 2: We know that the volume of a triangular prism is equal to B × l.

These will also be our first two sides, so s1 = 4 and s2 = 7. In this example, the base area of the prism is 100 sq. An easy way to compute the lateral area for a right prism is LAph where LA is the lateral. The triangular prism contains 5 faces, 9 edges, and 6 vertices. Solution: 1.) Since the base of the prism is formed by a right triangle and we know the leg lengths of the triangle, we can use the legs as the base and height. The volume is (b) The lateral area is the surface area minus the area of the bases. In mathematics, a triangular prism is a three-dimensional solid shape with two identical ends connected by equal parallel lines. Find the surface area of the triangular prism. The lateral faces of the prism are formed by a rectangle with a length of 5.

Problem 1: The bases of a triangular prism are formed by a right triangle with leg lengths of 4 and 7.


 0 kommentar(er)
0 kommentar(er)
